Mixed-up Answers

Today, designing a jet requires costly high-speed wind tunnel experiments to determine how the atmosphere interacts with the shape of the plane. Meteorologists, meanwhile, can’t fully predict the movement of heat from the ocean into a hurricane, leading to forecasts that can be dangerously uncertain.

But a recent breakthrough by University of Maryland mathematicians provides the first evidence that the laws of turbulence at the heart of such problems can be understood. It demonstrates the possibility that engineers might one day bypass the need for building costly models when designing the planes of the future, while weather forecasters could eliminate guesswork and better predict hurricane path possibilities.

Mathematics Professor Jacob Bedrossian, Samuel Punshon-Smith Ph.D. ’17 and postdoctoral researcher Alex Blumenthal developed the first rigorous mathematical proof for a fundamental law of turbulence known as Batchelor’s law, which helps explain how chemical concentrations and temperature variations distribute themselves throughout a fluid. It was presented last month at a meeting of the Society for Industrial and Applied Mathematics.

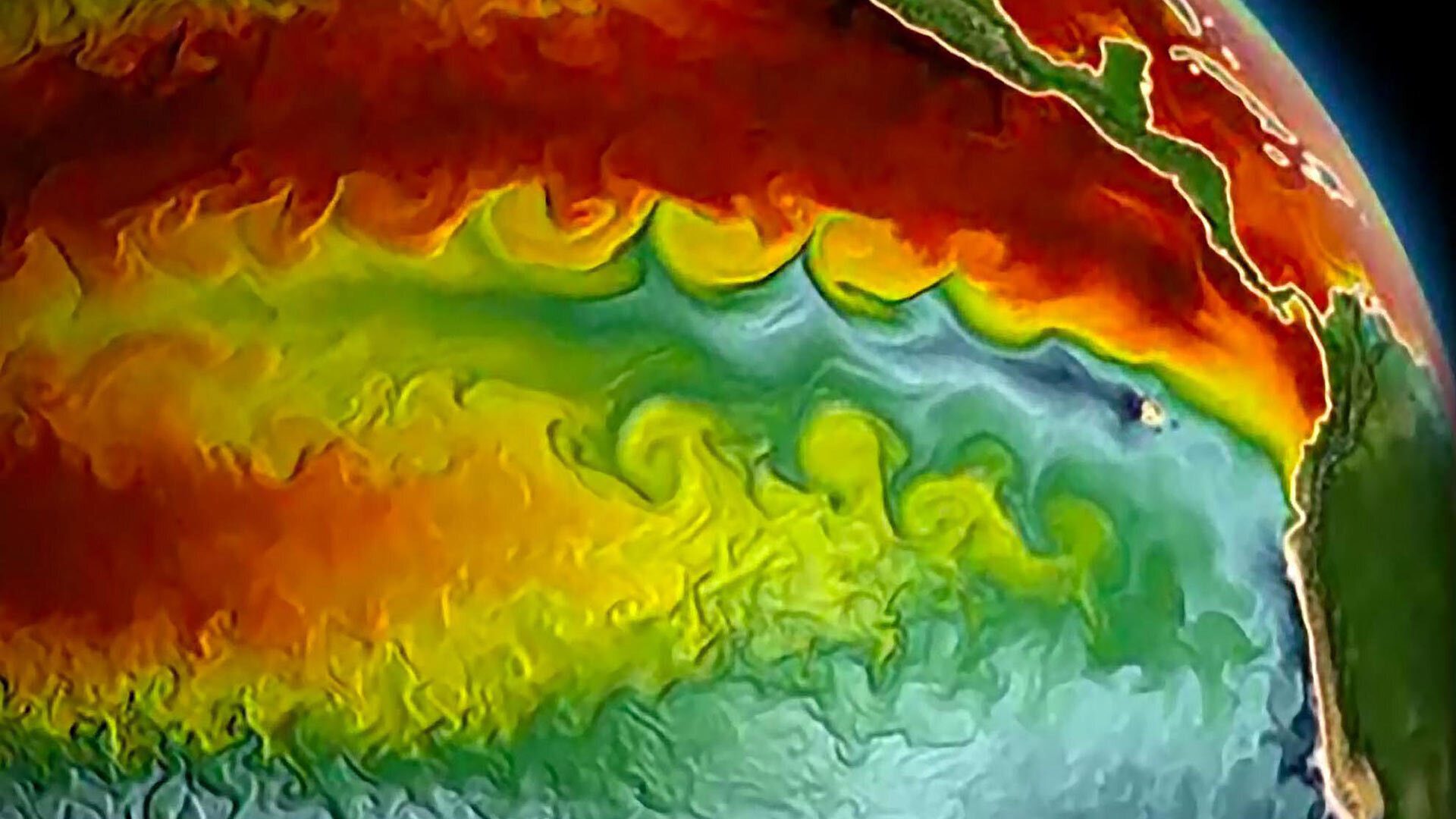
While mathematical equations can describe the laws of physics, their underlying principles aren’t always supported by detailed mathematical proofs. One area of physics long considered too challenging to explain with rigorous mathematics is turbulence. Seen in ocean surf, billowing clouds, aircraft vapor trails and wakes behind boats, turbulence is the chaotic movement of fluids and gases that includes seemingly random changes in pressure and velocity.

Turbulence is the reason that the equations describing fluid flow—known as the Navier-Stokes equations—are so hard to solve that a million-dollar reward will go to the first person or team to prove them mathematically. To understand fluid flow, scientists must first understand turbulence.

“It should be possible to look at a physical system and understand mathematically if a given physical law is true,” said Bedrossian. “We believe our proof provides the foundation for understanding why Batchelor’s law, a key law of turbulence, is true in a way that no theoretical physics work has done so far. This work could help clarify some of the variations seen in turbulence experiments and predict the settings where Batchelor’s law applies as well as where it doesn’t.”

Since its introduction in 1959, physicists have debated the validity and scope of Batchelor’s law, which has relevance to many aspects of everyday life. For example, stirring cream into coffee or oil into gasoline creates a large swirl with small swirls branching off of it and even smaller ones branching off of those. As the fluids mix, the swirls grow smaller and the level of detail changes at each scale. Batchelor’s law predicts the detail of those swirls at different scales.

The law also plays a role in such things as river water blending with saltwater as it flows into the ocean and warm Gulf Stream water combining with cooler water as it flows north. Over the years, many important contributions have helped illuminate Batchelor’s law, including work at UMD by Distinguished University Professors Thomas Antonsen and Edward Ott. However, a complete mathematical proof remained elusive.

“But we were able to crack the problem by combining expertise from multiple fields,” said Bedrossian, who has a joint appointment in UMD’s Center for Scientific Computation and Mathematical Modeling.

An expert in partial differential equations, Bedrossian brought in two UMD postdoctoral researchers who are experts in three other areas to help him solve the problem. Punshon-Smith, now the Prager Assistant Professor at Brown University, is an expert in probability. Blumenthal is an expert in dynamical systems and ergodic theory, a branch of mathematics that includes what is commonly known as chaos theory.

“The way the problem has been approached is indeed creative and innovative,” said Vladimir Sverak, a professor of mathematics at the University of Minnesota who was not involved in the work. “Sometimes the method of proof can be even more important than the proof itself. It is likely that ideas from the papers by Professor Bedrossian and his co-authors will be very useful in future research.”

The new level of collaboration that the team brought to this issue sets the stage for developing mathematical proofs to explain other unproven laws of turbulence.

“If this proof is all we achieve, I think we’ve accomplished something,” Bedrossian said. “But I’m hopeful that this is a warmup and that this opens a door to saying, ‘Yes, we can prove universality laws of turbulence, and they are not beyond the realm of mathematics.’”